Cavity Kerr Effect

S. Gupta, K. L. Moore, K. W. Murch, and D. M. Stamper-Kurn, Cavity nonlinear optics at low photon numbers from collective atomic motion, quant-ph/0706.1052 (June 2007)

Nonlinear optical phenomena typically occur at high optical intensities, as conventional materials mediate only weak coupling between photons. Under the condition of strong coupling, atomic saturation leads a host of nonlinear effects. In our experiment collective atomic motion mediates the coupling between photons. The long lived motional coherence of ultracold gasses permits nonlinear optical phenomena to occur when the average number of photons in the cavity is far less than one.

When atoms are located at the gradient of the probe standing wave field, forces from the probe potential cause a spatial shift of the atoms, changing the coupling, and thereby the total index of refraction. This is analogous to the Kerr effect in solids. This Kerr-effect leads to asymmetric and bistable spectra of the cavity, and is equivalent to they system of a damped, driven, nonlinear oscillator.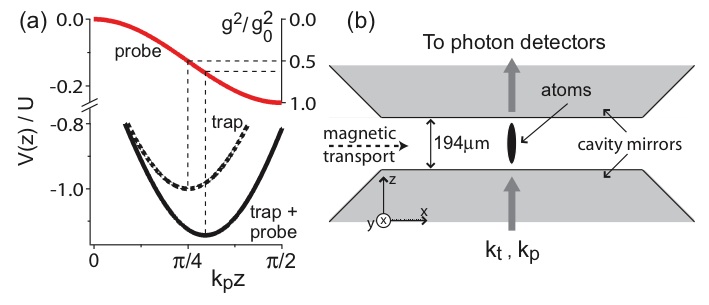

The interaction of the atoms with the probe light depends on the position of the atoms, since they experience different intensities of the probe depending on where in the standing wave they are located. If the atoms are distributed evenly throughout the standing wave, the average interaction is simply half of the maximum.

Consider, for example a sample of atoms which are harmonically trapped at a location which is located at the inflection point of the probe intensity. Here, atoms experience a force, due to the gradient of the AC stark shift potential, which is proportional to the intensity of the probe light in the cavity. The effect of this force is to cause a spatial shift in the position of the trap minimum, and thereby the interaction of the atoms with the probe.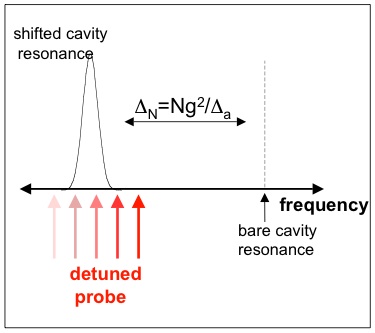

We probe the atom cavity system with probe light far detuned from the atomic resonance, in this limit, each atom can be considered as a small piece of refractive medium, changing the index of refraction inside the cavity. Here, the presence of N atoms shifts the resonance of the atom cavity system by an amount

{$\Delta_N = N g^2/\Delta_a$}

Accounting for how the probe changes the spatial position of the atoms, and thereby the coupling, {$g$}. {$\Delta_N$} is then partially proportional to intensity of the probe.

{$\Delta_N =\frac{ N g^2}{\Delta_a} (1 + \epsilon \bar{n})$}

Where, {$\epsilon $} depends on the parameters of the system, and {$\bar{n}$}, the average number of photons in the cavity characterizes the intracavity intensity. This indicates that the atom-cavity linecenter depends on the probe intensity.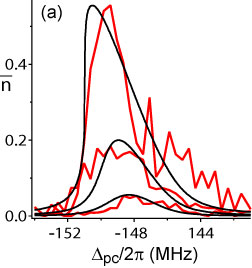

We study this effect by sweeping the probe frequency across the cavity resonance. For probe light which is red-detuned from the atomic resonance, the resulting position shift moves atoms to regions of higher coupling, and shifts the cavity resonance further to the left (i.e. larger {$|\Delta_N|$}). When the probe light is swept across the resonance, an asymmetric transmission is observed. Here, as the probe is swept from left to right, the increasing intensity causes the cavity line to shift increasingly to the left, resulting in the observed asymmetric spectra.

The cavity lineshape is described by a lorentzian who's line center depends on the intracavity intensity. For a probe of constant intensity {$\bar{n}_{max}$}, the resulting intensity is,

{$ \bar{n} = \frac{\bar{n}_{max}}{1+ (\frac{\Delta_{pc} - \Delta_N (1+\epsilon \bar{n})}{\kappa})^2} $}

Where {$\Delta_{pc}$} is the detuning of the probe frequency from the bare cavity resonance. The intracavity intensity {$\bar{n}$} is now described by a cubic function, for which we may in some cases have three real solutions, corresponding to a situation where for a constant drive, there are three possible intracavity intensities which may take place.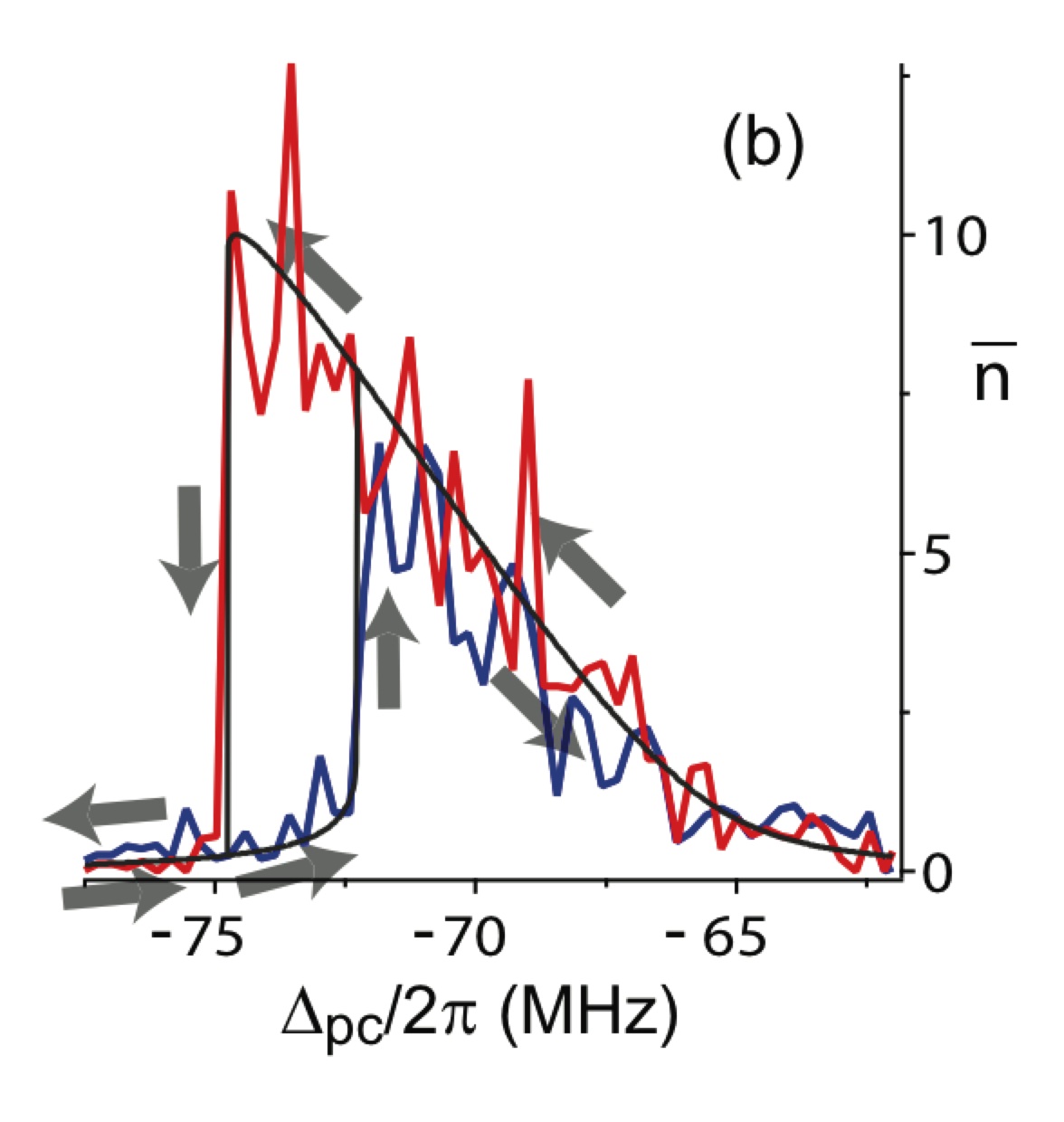

In practice, though there are three possible real solutions to the intracavity intensity, only two of these solutions are stable. Tuning the parameters of our system to this region of bistability we observe starkly different transmissions depending on whether the sweep of the probe was from right to left or from left to right.

In contrast to previous work in Cavity QED systems which observed absorptive bistability1-3, the dispersive bistabilty observed here results from motion of the atomic medium, and not from an atomic saturation based nonlinearity.